| Copyright – D. McAuley, GlobalRPh Inc., All Rights Reserved. | |||||||||||||||||||||||||
| This initial program provides some general dosage guidelines based on population averages for the Michaelis-Menten parameters (Km and Vmax). The recommendations do not take into account the following: (1) existence of interacting drugs (3) inter-patient variability (3) existing disease states which may significantly alter the eventual therapeutic dose.
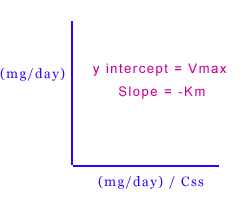
The program uses a maintenance dose of 4 to 6 mg/kg for all adults < 60 years old. The elderly (defined as age>60) on the other hand, exhibit a saturation of metabolism at dosages that are usually 20 % lower than in younger patients. For this reason, the program recommends initial dosages of 3-4 mg/kg in this age group. (Note: many studies have found only slight variations in Km with regards to age, however, Vmax has been found to decline steadily with age–most notably after the 6th decade). The program also calculates an “adjusted dose” for reported levels when the serum albumin level is < 4.5 g/dl. The program accomplishes this by utilizing the Sheiner-Tozer equation: C adj = Creported /(0.2 x serum albumin) + 0.1. In order to simplify the program, all dosages are based on the adjusted body weight. This is especially important in obese patients where the utilization of total body weight would result in an over-estimation of dosage. Projected loading doses required to increase sub-therapeutic concentrations to the therapeutic range are determined by using the following equation: Cadj = (Concentration desired – Concentration observed) x Vd, where Vd = 0.7 x adjusted body weight. The usual range for Vd is 0.52 to 0.78 L/kg. I must stress again the general nature of this program. Significant inter-patient variability may exist for many of the pharmacokinetic parameters. Here are some reported ranges: Vd: 0.52 – 0.78 L/kg Vmax: 6 – 8 mg/kg/day Km: 5.4 – 6.8 mg/L. To ensure greater accuracy and appropriateness of dosing, individual determination of Vmax and Km values for each patient based on steady state levels is recommended. The maintenance dose can then be calculated using the following equation:
|
|||||||||||||||||||||||||
Other Equations |
|||||||||||||||||||||||||
|
It is important to remember that since phenytoin’s elimination is a saturable process, and clearance decreases with increasing concentrations, the steady state concentration is NOT proportional to the maintenance dose (e.g. a non-linear relationship exists). Also, the half-life has little value in estimating the time to steady state. |
|||||||||||||||||||||||||
Additional Info |
|||||||||||||||||||||||||
| Note: program uses adjusted body weight for all calculations. Loading Dose (IV): 10 – 20 mg/kg . Recommended infusion rate for adults: 40-50 mg/min. Elderly (>65): Recommended infusion rate: 20-25 mg/min. Oral loading: Give in 3 to 4 divided doses at q2h intervals. (Divided doses increase bioavailability as well as decrease potential for GI side effects such as N&V). The maximum single oral dose should not exceed 400 mg in order to minimize GI side effects and also increase absorption (decrease likelihood of concretions). Sampling: 18 to 24 hours after the loading dose, and then every 5 to 7 days to assess trend. Average time to steady state: 10-14 days. Half-life: 7 to 42 hours (average = 24 hours). Conversion to once daily dosing: Consider only after a divided dose regimen on extended phenytoin capsules is established. (Only extended release Dilantin caps are recommended for once daily administration.) A patient should never receive a once daily dose of elixir or injection as maintenance. When do you start the maintenance dose? The maintenance dose is started 18-24 hours after the loading dose. Capsules/injection= 92% phenytoin (sodium salt). Elixir/tabs=100% phenytoin. Equation used to estimate the dose required to increase current level to normal range if subtherapeutic: = [0.7 x IBW x (15 – current level) ] / 0.92* * (if capsules/injection used) Adjusted phenytoin concentration if low serum albumin = measured total concentration / [ (0.2 x albumin) + 0.1]
|
|||||||||||||||||||||||||
Cautions |
|||||||||||||||||||||||||
| (1) Always remember that because phenytoin’s elimination is dose-dependent (“capacity limited”), that small increases in dosage can produce disproportionate increases in serum levels (possibly 3 to 4 fold).
(2) Never assume a linear relationship exists between steady state concentrations and the dosage given. |
|||||||||||||||||||||||||
References |
|||||||||||||||||||||||||
|
|||||||||||||||||||||||||
Don’t forget to check out the extensive health section…. |